The demand curve can be written as Q=20-.8P or as P=25-1.25Q. For purposes of drawing graphs as we conventionally do them the latter representation is more useful. The supply curve can also be written two ways: Q=-4+.4P or P=10+2.5Q. For graphing purposes the latter is easier to work with.

Setting quantity demanded equal to quantity supplied we get

20-.8P=-4+.4P

24=1.2P

Substitute P=20 back into the demand curve to get Q=20-.8(20)=4. The equilibrium price and quantity are shown in the diagram below.

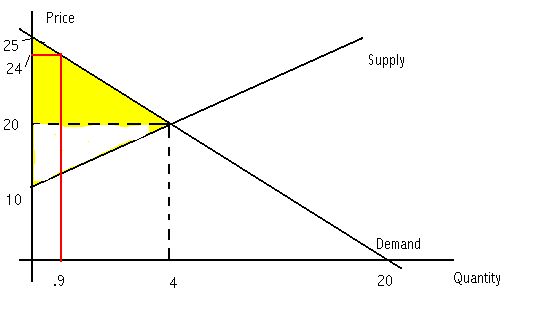
1. Consumer surplus is the area under the demand curve but above the going price. In the diagram consumer surplus corresponds to the area of the yellow triangle. The area of a right triangle is found from formula area=(1/2)*base*height. In this example the base is 4, the height is 25-20=5. Therefore consumer surplus is .5*4*5=10.
2. There are ten identical consumers. If total market demand at P=$20 is 4 then each consumer must have purchased .4 units. Similarly, at a price of $24 the total quantity demanded is .9, therefore each consumer must have purchased .09. At a price of $25 no one buys anything. Applying the notion of consumer surplus to the individual, gives us CS=.5*(.4)*(25-20)=1. The conclusion is that each buyer has a consumer surplus of 1. That is the most any one of them would be willing to pay to become a member.
3. Having to pay a ‘membership fee’ will not change any individual’s final demand for the product. Why? At a price of $25 no one would purchase anything. When the price falls to $24 then buyers will purchase .9 units. At the market clearing price of $20, those who would have paid $24 for the first .9 units feel as though they got a bargain. They ‘saved’ .9*(24-20)=$3.60 by virtue of the fact that they need only pay the market price. Suppose we now inform this group that although they only have to pay $20/unit for their purchases of .9 units they must also pool their resources and pay a membership fee of $3.60. Their total expense is 20*.9+3.60=$21.60. As far as this first group of consumers is concerned this is no different than the $24/unit they would have paid for the first .9 units in the first place, .9*24=$21.60.