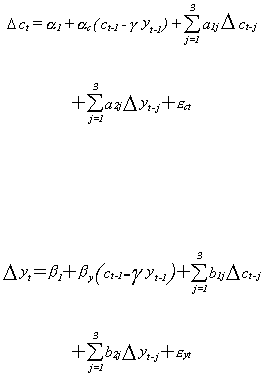Temple University
Department of Economics

Econometrics II
Spurious Regressions, Unit Roots and Cointegration

From the web site you will fetch a file titled Y&NOND.DAT. It contains a column for the year (1947-1980) and a column for the quarter (1-4). The third column is income and the fourth is nondurable consumption. The data is in billions of 1982 dollars and has been deseasonalized.

1. Graph both income and nondurable consumption. Do the series appear to you to be stationary?

2. Take natural logs of both series; call them y and c respectively. Graph the transformed series. Do the new series appear to be stationary?

3. Estimate the parameters of

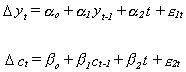
The intercept allows for drift in the process and the time variable allows for a deterministic trend. Use the regression results to test the following hypotheses:
a. There is neither drift nor deterministic trend.
b. There is a unit root in each of income and consumption.

4. Estimate the parameters of

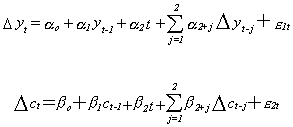
Using this augmented Dickey-Fuller framework, comment on the stationarity of the Dc_t and Dy_t series. On the basis of your work in this problem set, do you now believe that you may have run a spurious regression in an earlier homework assignment?


5. Estimate the parameters of the long run relationship

Call the residuals from this estimation and fit the model

Is there a unit root in the residual series? What do you conclude about the cointegration of y_t and c_t?

6. Regardless of what you may have concluded in question 5, estimate the error correction models

7. What are the long run and short run propensities to consume? Are these results consistent with those found by Friedman in his classic work on consumption?